Reader TTS

ABSTRACT Phase competition underlies many remarkable and technologically important phenomena in transition metal oxides. Vanadium dioxide (VO2) exhibits a first-order metal–insulator

transition (MIT) near room temperature, where conductivity is suppressed and the lattice changes from tetragonal to monoclinic on cooling. Ongoing attempts to explain this coupled structural

and electronic transition begin with two alternative starting points: a Peierls MIT driven by instabilities in electron–lattice dynamics and a Mott MIT where strong electron–electron

correlations drive charge localization1,2,3,4,5,6,7,8,9,10. A key missing piece of the VO2 puzzle is the role of lattice vibrations. Moreover, a comprehensive thermodynamic treatment must

integrate both entropic and energetic aspects of the transition. Here we report that the entropy driving the MIT in VO2 is dominated by strongly anharmonic phonons rather than electronic

contributions, and provide a direct determination of phonon dispersions. Our _ab initio_ calculations identify softer bonding in the tetragonal phase, relative to the monoclinic phase, as

the origin of the large vibrational entropy stabilizing the metallic rutile phase. They further reveal how a balance between higher entropy in the metal and orbital-driven lower energy in

the insulator fully describes the thermodynamic forces controlling the MIT. Our study illustrates the critical role of anharmonic lattice dynamics in metal oxide phase competition, and

provides guidance for the predictive design of new materials. Access through your institution Buy or subscribe This is a preview of subscription content, access via your institution ACCESS

OPTIONS Access through your institution Subscribe to this journal Receive 51 print issues and online access $199.00 per year only $3.90 per issue Learn more Buy this article * Purchase on

SpringerLink * Instant access to full article PDF Buy now Prices may be subject to local taxes which are calculated during checkout ADDITIONAL ACCESS OPTIONS: * Log in * Learn about

institutional subscriptions * Read our FAQs * Contact customer support SIMILAR CONTENT BEING VIEWED BY OTHERS ORBITAL-SELECTIVE MOTT AND PEIERLS TRANSITION IN H_X_VO2 Article Open access 23

September 2022 MAGNETIC-FIELD-INDUCED INSULATOR–METAL TRANSITION IN W-DOPED VO2 AT 500 T Article Open access 17 July 2020 INSULATOR-TO-METAL TRANSITION IN ULTRATHIN RUTILE VO2/TIO2(001)

Article Open access 12 July 2022 REFERENCES * Goodenough, J. B. The two components of crystallographic transition in VO2 . _J. Solid State Chem._ 3, 490–500 (1971) ADS  CAS  Google Scholar 

* Whittaker, L., Patridge, C. J. & Banerjee, S. Microscopic and nanoscale perspective of the metal-insulator phase transitions of VO2: some new twists to an old tale. _J. Phys. Chem.

Lett._ 2, 745–758 (2011) CAS  Google Scholar  * Eyert, V. The metal-insulator transitions of VO2: a band theoretical approach. _Ann. Phys. (Leipzig)_ 11, 650–704 (2002) ADS  CAS  MATH 

Google Scholar  * Park, J. H. et al. Measurement of a solid-state triple point at the metal-insulator transition in VO2 . _Nature_ 500, 431–434 (2013) ADS  CAS  PubMed  Google Scholar  *

Zylbersztejn, A. & Mott, N. F. Metal-insulator transition in vanadium dioxide. _Phys. Rev. B_ 11, 4383–4395 (1975) ADS  CAS  Google Scholar  * Hearn, C. J. Phonon softening and

metal-insulator transition in VO2 . _J. Phys. C_ 5, 1317–1334 (1972) ADS  CAS  Google Scholar  * Wentzcovitch, R. M., Schulz, W. W. & Allen, P. B. VO2 - Peierls or Mott-Hubbard - a view

from band theory. _Phys. Rev. Lett._ 72, 3389–3392 (1994) ADS  CAS  PubMed  Google Scholar  * Rice, T. M. et al. Comment and reply on ‘VO2 - Peierls or Mott-Hubbard - a view from band

theory’. _Phys. Rev. Lett._ 73, 3042–3043 (1994) ADS  CAS  PubMed  Google Scholar  * Berglund, C. N. & Guggenheim, H. J. Electronic properties of VO2 near the semiconductor-metal

transition. _Phys. Rev._ 185, 1022–1033 (1969) ADS  CAS  Google Scholar  * Nakano, M. et al. Collective bulk carrier delocalization driven by electrostatic surface charge accumulation.

_Nature_ 487, 459–462 (2012) ADS  CAS  PubMed  Google Scholar  * Aetukuri, N. B. et al. Control of the metal-insulator transition in vanadium dioxide by modifying orbital occupancy. _Nature

Phys._ 9, 661–666 (2013) ADS  CAS  Google Scholar  * Jeong, J. et al. Suppression of metal-insulator transition in VO2 by electric field-induced oxygen vacancy formation. _Science_ 339,

1402–1405 (2013) ADS  CAS  PubMed  Google Scholar  * Srivastava, R. & Chase, L. L. Raman spectrum of semiconducting and metallic VO2 . _Phys. Rev. Lett._ 27, 727–730 (1971) ADS  CAS 

Google Scholar  * Terauchi, H. & Cohen, J. B. Diffuse X-ray-scattering due to lattice instability near the metal-semiconductor transition in VO2 . _Phys. Rev. B_ 17, 2494–2496 (1978) ADS

  CAS  Google Scholar  * McWhan, D. B., Marezio, M., Remeika, J. P. & Dernier, P. D. X-ray-diffraction study of metallic VO2 . _Phys. Rev. B_ 10, 490–495 (1974) ADS  CAS  Google Scholar

  * Maurer, D., Leue, A., Heichele, R. & Müller, V. Elastic behavior near the metal-insulator transition of VO2 . _Phys. Rev. B_ 60, 13249–13252 (1999) ADS  CAS  Google Scholar  * Pynn,

R., Axe, J. D. & Raccah, P. M. Structural fluctuations in NbO2 at high temperatures. _Phys. Rev. B_ 17, 2196–2205 (1978) ADS  CAS  Google Scholar  * Gervais, F. & Kress, W.

Lattice-dynamics of oxides with rutile structure and instabilities at the metal-semiconductor phase-transitions of NbO2 and VO2 . _Phys. Rev. B_ 31, 4809–4814 (1985) ADS  CAS  Google Scholar

  * Kim, S., Kim, K., Kang, C. J. & Min, B. I. Correlation-assisted phonon softening and the orbital-selective Peierls transition in VO2 . _Phys. Rev. B_ 87, 195106 (2013) ADS  Google

Scholar  * Pintchovski, F., Glaunsinger, W. S. & Navrotsky, A. Experimental study of electronic and lattice contributions to VO2 transition. _J. Phys. Chem. Solids_ 39, 941–949 (1978)

ADS  CAS  Google Scholar  * Qu, B. Y., He, H. Y. & Pan, B. C. The dynamical process of the phase transition from VO2(M) to VO2(R). _J. Appl. Phys._ 110, 113517 (2011) ADS  Google Scholar

  * Hellman, O., Steneteg, P., Abrikosov, I. A. & Simak, S. I. Temperature dependent effective potential method for accurate free energy calculations of solids. _Phys. Rev. B_ 87, 104111

(2013) ADS  Google Scholar  * Comes, R., Felix, P., Lambert, M. & Villeneuve, G. Metal to insulator phase-transition in V0,90Nb0,10O2 explained by local pairing of vanadium atoms. _Acta

Crystallogr. A_ 30, 55–60 (1974) ADS  Google Scholar  * Traylor, J. G., Smith, H. G., Nicklow, R. M. & Wilkinson, M. K. Lattice dynamics of rutile. _Phys. Rev. B_ 3, 3457–3472 (1971)

ADS  Google Scholar  * Haverkort, M. W. et al. Orbital-assisted metal-insulator transition in VO2 . _Phys. Rev. Lett._ 95, 196404 (2005) ADS  CAS  PubMed  Google Scholar  * Khomskii, D. I.

& Mizokawa, T. Orbitally induced Peierls state in spinels. _Phys. Rev. Lett._ 94, 156402 (2005) ADS  CAS  PubMed  Google Scholar  * Tomczak, J. M., Aryasetiawan, F. & Biermann, S.

Effective bandstructure in the insulating phase versus strong dynamical correlations in metallic VO2 . _Phys. Rev. B_ 78, 115103 (2008) ADS  Google Scholar  * Weber, C. et al. Vanadium

dioxide: a Peierls-Mott insulator stable against disorder. _Phys. Rev. Lett._ 108, 256402 (2012) ADS  PubMed  Google Scholar  * Yuan, X., Zhang, Y. B., Abtew, T. A., Zhang, P. H. &

Zhang, W. Q. VO2: Orbital competition, magnetism, and phase stability. _Phys. Rev. B_ 86, 235103 (2012) ADS  Google Scholar  * Eyert, V. VO2: a novel view from band theory. _Phys. Rev.

Lett._ 107, 016401 (2011) ADS  CAS  PubMed  Google Scholar  * Abernathy, D. L. et al. Design and operation of the wide angular-range chopper spectrometer ARCS at the Spallation Neutron

Source. _Rev. Sci. Instrum._ 83, 015114 (2012) ADS  CAS  PubMed  Google Scholar  * Squires, G. L. _Introduction to the Theory of Neutron Scattering_ 30–60 (Cambridge Univ. Press, 1978)

Google Scholar  * Manley, M. E. et al. Vibrational and electronic entropy of beta-cerium and gamma-cerium measured by inelastic neutron scattering. _Phys. Rev. B_ 65, 144111 (2002) ADS 

Google Scholar  * Karapetrova, E., Ice, G., Tischler, J., Hong, H. & Zschack, P. Design and performance of the 33-BM beamline at the Advanced Photon Source. _Nucl. Instrum. Methods_ 649,

52–54 (2011) ADS  CAS  Google Scholar  * Comes, R., Lambert, M. & Guinier, A. Linear disorder in crystals (silicon, quartz and ferroelectric perovskites). _Acta Crystallogr. A_ 26,

244–254 (1970) ADS  CAS  Google Scholar  * Lovesey, S. W. _The Theory of Neutron Scattering from Condensed Matter_ 296–303 (Oxford Univ. Press, 1985) Google Scholar  * Kresse, G. &

Hafner, J. _Ab initio_ molecular dynamics for liquid metals. _Phys. Rev. B_ 47, 558 (1993) ADS  CAS  Google Scholar  * Kresse, G. & Furthmuller, J. Efficient iterative schemes for _ab

initio_ total-energy calculations using a plane-wave basis set. _Phys. Rev. B_ 54, 11169 (1996) ADS  CAS  Google Scholar  * Blöchl, P. E. Projector augmented-wave method. _Phys. Rev. B_ 50,

17953 (1994) ADS  Google Scholar  * Kresse, G. & Joubert, D. From ultrasoft pseudopotentials to the projector augmented-wave method. _Phys. Rev. B_ 59, 1758–1775 (1999) ADS  CAS  Google

Scholar  * Longo, J. M. & Kierkegaard, P. A refinement of the structure of VO2 . _Acta Chem. Scand._ 24, 420–426 (1970) CAS  Google Scholar  * Togo, A., Oba, F. & Tanaka, I.

First-principles calculations of the ferroelastic transition between rutile-type and CaCl2-type SiO2 at high pressure. _Phys. Rev. B_ 78, 134106 (2008) ADS  Google Scholar  * Perdew, J. P.,

Burke, K. & Ernzerhof, M. Generalized gradient approximation made simple. _Phys. Rev. Lett._ 77, 3865–3868 (1996) ADS  CAS  PubMed  Google Scholar  * Liechtenstein, A. I., Anisimov, V.

I. & Zaanen, J. Density-functional theory and strong interactions: orbital ordering in Mott-Hubbard insulators. _Phys. Rev. B_ 52, R5467–R5470 (1995) ADS  CAS  Google Scholar  * Liebsch,

A., Ishida, H. & Bihlmayer, G. Coulomb correlations and orbital polarization in the metal-insulator transition of VO2 . _Phys. Rev. B_ 71, 085109 (2005) ADS  Google Scholar  * Heyd, J.,

Scuseria, G. E. & Ernzerhof, M. Hybrid functionals based on a screened Coulomb potential. _J. Chem. Phys._ 118, 8207 (2003); erratum. 124, 219906 (2006) ADS  CAS  Google Scholar  *

Sugiyama, K. & Takeuchi, Y. The crystal structure of rutile as a function of temperature up to 1600°C. _Z. Kristallogr._ 194, 305–313 (1991) CAS  Google Scholar  Download references

ACKNOWLEDGEMENTS Research by J.D.B., O.D., M.E.M., E.D.S., L.A.B. and R.J.M. was supported by the US Department of Energy (DOE), Basic Energy Sciences (BES), Materials Sciences and

Engineering Division (MSED). Research by J.H. was supported by the Center for Accelerating Materials Modeling, funded by the US DOE, BES, MSED. Experimental work by C.W.L. was sponsored by

the Laboratory Directed Research and Development Program of ORNL (Principal Investigator, O.D.). Research by D.L.A. at the Spallation Neutron Source and J.Z.T., A.H.S. and B.M.L. at the

Advanced Photon Source (APS), Argonne National Laboratory (ANL), was supported by the US DOE, BES, Scientific User Facilities Division. We thank A. Tselev, S. Nagler, A. Banerjee, H.

Krakauer and V. Cooper for interesting discussions on VO2. Inelastic neutron scattering measurements were performed using the ARCS facility at the ORNL Spallation Neutron Source, which is

sponsored by the Scientific User Facilities Division, Office of Basic Energy Sciences, US Department of Energy. We thank J. Niedziela for help with the sample environment at ARCS. IXS

measurements were performed using the X-ray Operations and Research (XOR) beamline 30-ID (HERIX) at the APS. Diffuse X-ray scattering measurements were performed using the XOR beamline

33-BM-C at the APS. We thank J. Karapetrova and C. Schleputz for assistance in setting up experiments at UNICAT. Use of the APS, an Office of Science User Facility operated for the US DOE

Office of Science by ANL, was supported by the US DOE under contract no. DE-AC02-06CH11357. Theoretical calculations were performed using resources of the National Energy Research Scientific

Computing Center, a DOE Office of Science User Facility supported by the Office of Science of the US Department of Energy under contract no. DE-AC02-05CH11231. We thank O. Hellman for

providing the temperature-dependent effective potential software and assistance. AUTHOR INFORMATION Author notes * John D. Budai and Jiawang Hong: These authors contributed equally to this

work. AUTHORS AND AFFILIATIONS * Materials Science and Technology Division, Oak Ridge National Laboratory, Oak Ridge, 37831, Tennessee, USA John D. Budai, Jiawang Hong, Michael E. Manley, 

Eliot D. Specht, Chen W. Li, Lynn A. Boatner & Olivier Delaire * Advanced Photon Source, Argonne National Laboratory, Argonne, 60439, Illinois, USA Jonathan Z. Tischler, Ayman H. Said 

& Bogdan M. Leu * Quantum Condensed Matter Division, Oak Ridge National Laboratory, Oak Ridge, 37831, Tennessee, USA Douglas L. Abernathy * Neutron Sciences Directorate, Oak Ridge

National Laboratory, Oak Ridge, 37831, Tennessee, USA Robert J. McQueeney Authors * John D. Budai View author publications You can also search for this author inPubMed Google Scholar *

Jiawang Hong View author publications You can also search for this author inPubMed Google Scholar * Michael E. Manley View author publications You can also search for this author inPubMed 

Google Scholar * Eliot D. Specht View author publications You can also search for this author inPubMed Google Scholar * Chen W. Li View author publications You can also search for this

author inPubMed Google Scholar * Jonathan Z. Tischler View author publications You can also search for this author inPubMed Google Scholar * Douglas L. Abernathy View author publications You

can also search for this author inPubMed Google Scholar * Ayman H. Said View author publications You can also search for this author inPubMed Google Scholar * Bogdan M. Leu View author

publications You can also search for this author inPubMed Google Scholar * Lynn A. Boatner View author publications You can also search for this author inPubMed Google Scholar * Robert J.

McQueeney View author publications You can also search for this author inPubMed Google Scholar * Olivier Delaire View author publications You can also search for this author inPubMed Google

Scholar CONTRIBUTIONS This project included significant contributions from many researchers and all authors participated in scientific discussions. J.D.B. (experiment) and O.D. (experiment

and calculations) designed this research project. L.A.B. synthesized single-crystal samples. J.H. and O.D. performed the theoretical calculations with analysis. M.E.M., C.W.L., J.D.B., O.D.

and D.L.A. performed the INS measurements and analysis. E.D.S., J.D.B., O.D., C.W.L. and J.Z.T. performed the diffuse X-ray scattering measurements and analysis. J.D.B., M.E.M., O.D.,

C.W.L., A.H.S., B.M.L., J.Z.T. and R.J.M. performed the IXS measurements and analysis. O.D., J.D.B., M.E.M., E.D.S. and J.H. wrote the manuscript with assistance from C.W.L. CORRESPONDING

AUTHORS Correspondence to John D. Budai or Olivier Delaire. ETHICS DECLARATIONS COMPETING INTERESTS The authors declare no competing financial interests. EXTENDED DATA FIGURES AND TABLES

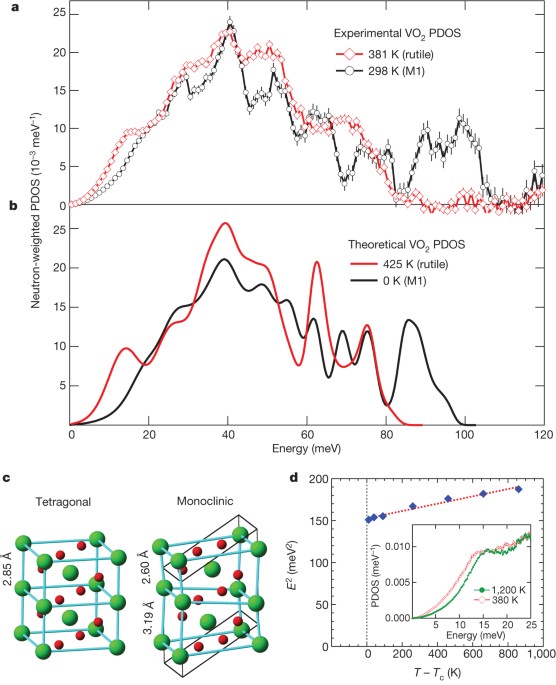
EXTENDED DATA FIGURE 1 TEMPERATURE-DEPENDENT SCATTERING FUNCTION _S_(_E_). Data come from inelastic powder neutron scattering measurements integrated over _Q_ values up to ∼6 Å−1, obtained

using an incident neutron energy of 30 meV, and show gradual softening of the low-energy phonons and abrupt disappearance of these modes across the transition at temperature _T_c. EXTENDED

DATA FIGURE 2 OPTICAL PHOTOGRAPH OF RECTANGULAR VO2 SINGLE CRYSTAL MOUNTED ON COPPER POST. Crystal dimensions are 0.25 mm × 0.25 mm × ∼4 mm. Unlike larger crystals, small crystals such as

this did not show sample cracking while thermally cycling through the MIT. EXTENDED DATA FIGURE 3 INTEGRATED FIRST-ORDER TDS INTENSITY FOR A _H_ + _K_ + _L_ = 8 SHEET NEAR (4.5 0 3.5) USING

DIFFUSE X-RAY SCATTERING MEASUREMENTS FROM THE APS-33BM BEAMLINE, COMPARED WITH THE THERMAL OCCUPATION FACTOR FOR PHONONS. EXTENDED DATA FIGURE 4 IXS ENERGY SCAN. Data (filled blue circles)

are for a transverse acoustic phonon in the rutile phase measured at an M point in reciprocal space, _Q_ = (0.5, 3.5, 0), at a temperature of 810 K. The solid red line is a fit using the

expression for a damped harmonic oscillator. EXTENDED DATA FIGURE 5 IXS MEASUREMENTS FOR INDIVIDUAL PHONON BRANCHES. Schematic at left shows total scattering vectors, _Q_LA and _Q_TA, for

separate measurements of longitudinal and transverse phonons with wavevector _Q_ = (0, 0, _ζ_) (_ζ_ ≈ 0.3) along the Γ–Z symmetry direction. Plot at right shows experimental energy scan at

_Q_TA = (0, 4, 0.3) corresponding to the transverse acoustic branch. The line shape is well fitted by a strongly damped harmonic model, and the large anharmonic linewidth corresponds to a

very short phonon lifetime. EXTENDED DATA FIGURE 6 CALCULATED PHONON DISPERSIONS WITH HARMONIC APPROXIMATIONS. Data calculated at 0 K with PBE (A) and PBE+_U_ (B). Negative energies

correspond to unstable modes with imaginary frequencies which are unphysical. EXTENDED DATA FIGURE 7 DFT FROZEN-PHONON POTENTIAL ENERGY CURVES (PER ATOM). Blue curves are parabolic fits and

magenta fits include quadratic as well as quartic terms. Insets show the displacement patterns of the modes. Black dots represent DFT total energy as a function of _u_Vmax, the maximum

displacement of a V atom in the phonon mode. Vanadium and oxygen atoms are depicted by green and red, respectively. SUPPLEMENTARY INFORMATION THREE-DIMENSIONAL THERMAL DIFFUSE SCATTERING

(TDS) X-RAY MEASUREMENTS Temperature-dependent x-ray diffuse scattering measurements from VO2 single crystals were measured using an area detector as the sample was rotated. The reciprocal

lattice vector was calculated for each pixel of the detector at each angle setting. Two-dimensional and three dimensional diffuse scattering maps were calculated by averaging the counts of

all pixels contained in each voxel of a regular 0.05 x 0.05 x 0.025 rutile reciprocal-lattice grid. The Supplementary Video illustrates that, as discussed in the article, the 3-D thermal

diffuse _H_+_K_+_L_=2_n_, _n_≠0) in reciprocal space. (MOV 1675 kb) POWERPOINT SLIDES POWERPOINT SLIDE FOR FIG. 1 POWERPOINT SLIDE FOR FIG. 2 POWERPOINT SLIDE FOR FIG. 3 POWERPOINT SLIDE FOR

FIG. 4 RIGHTS AND PERMISSIONS Reprints and permissions ABOUT THIS ARTICLE CITE THIS ARTICLE Budai, J., Hong, J., Manley, M. _et al._ Metallization of vanadium dioxide driven by large phonon

entropy. _Nature_ 515, 535–539 (2014). https://doi.org/10.1038/nature13865 Download citation * Received: 13 June 2014 * Accepted: 12 September 2014 * Published: 10 November 2014 * Issue

Date: 27 November 2014 * DOI: https://doi.org/10.1038/nature13865 SHARE THIS ARTICLE Anyone you share the following link with will be able to read this content: Get shareable link Sorry, a

shareable link is not currently available for this article. Copy to clipboard Provided by the Springer Nature SharedIt content-sharing initiative