Reader TTS

ABSTRACT Sixty years ago, Karplus and Luttinger pointed out that quantum particles moving on a lattice could acquire an anomalous transverse velocity in response to a force, providing an

explanation for the unusual Hall effect in ferromagnetic metals1. A striking manifestation of this transverse transport was then revealed in the quantum Hall effect2 where the plateaux

depicted by the Hall conductivity were attributed to a topological invariant characterizing the Bloch bands: the Chern number3. Until now, topological transport associated with non-zero

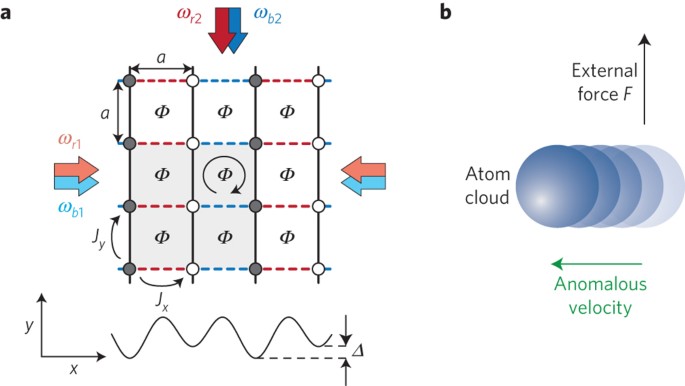
Chern numbers has only been observed in electronic systems2,4,5. Here we use the transverse deflection of an atomic cloud in response to an optical gradient to measure the Chern number of

artificially generated Hofstadter bands6. These topological bands are very flat and thus constitute good candidates for the realization of fractional Chern insulators7. Combining these

deflection measurements with the determination of the band populations, we obtain an experimental value for the Chern number of the lowest band _ν_exp = 0.99(5). This first Chern-number

measurement in a non-electronic system is facilitated by an all-optical artificial gauge field scheme, generating uniform flux in optical superlattices. Access through your institution Buy

or subscribe This is a preview of subscription content, access via your institution ACCESS OPTIONS Access through your institution Subscribe to this journal Receive 12 print issues and

online access $259.00 per year only $21.58 per issue Learn more Buy this article * Purchase on SpringerLink * Instant access to full article PDF Buy now Prices may be subject to local taxes

which are calculated during checkout ADDITIONAL ACCESS OPTIONS: * Log in * Learn about institutional subscriptions * Read our FAQs * Contact customer support SIMILAR CONTENT BEING VIEWED BY

OTHERS ENGINEERING NON-HERMITIAN SKIN EFFECT WITH BAND TOPOLOGY IN ULTRACOLD GASES Article Open access 13 October 2022 REALIZATION OF AN ANOMALOUS FLOQUET TOPOLOGICAL SYSTEM WITH ULTRACOLD

ATOMS Article 29 June 2020 TWO-DIMENSIONAL NON-HERMITIAN SKIN EFFECT IN AN ULTRACOLD FERMI GAS Article 08 January 2025 REFERENCES * Karplus, R. & Luttinger, J. M. Hall effect in

ferromagnetics. _Phys. Rev._ 95, 1154–1160 (1954). Article  ADS  Google Scholar  * Von Klitzing, K. The quantized Hall effect. _Rev. Mod. Phys._ 58, 519–531 (1986). Article  ADS  Google

Scholar  * Thouless, D. J., Kohmoto, M., Nightingale, M. P. & den Nijs, M. Quantized Hall conductance in a two-dimensional periodic potential. _Phys. Rev. Lett._ 49, 405–408 (1982). ADS

  Google Scholar  * Dean, C. R. et al. Hofstadter’s butterfly and the fractal quantum Hall effect in moiré superlattices. _Nature_ 497, 598–602 (2013). Article  ADS  Google Scholar  *

Ponomarenko, L. A. et al. Cloning of Dirac fermions in graphene superlattices. _Nature_ 497, 594–597 (2013). Article  ADS  Google Scholar  * Hofstadter, D. R. Energy levels and wave

functions of Bloch electrons in rational and irrational magnetic fields. _Phys. Rev. B_ 14, 2239–2249 (1976). Article  ADS  Google Scholar  * Parameswaran, S. A., Roy, R. & Sondhi, S. L.

Fractional quantum Hall physics in topological flat bands. _C. R. Phys._ 14, 816–839 (2013). Article  ADS  Google Scholar  * Goldman, N., Juzeliunas, G., Ohberg, P. & Spielman, I. B.

Light-induced gauge fields for ultracold atoms. Preprint at http://arXiv.org/abs/1308.6533 (2013) * Bermudez, A., Schaetz, T. & Porras, D. Synthetic gauge fields for vibrational

excitations of trapped ions. _Phys. Rev. Lett._ 107, 150501 (2011). Article  ADS  Google Scholar  * Rechtsman, M. C. et al. Photonic Floquet topological insulators. _Nature_ 496, 196–200

(2013). Article  ADS  Google Scholar  * Carusotto, I. & Ciuti, C. Quantum fluids of light. _Rev. Mod. Phys._ 85, 299–366 (2013). Article  ADS  Google Scholar  * Price, H. M. &

Cooper, N. R. Mapping the Berry curvature from semiclassical dynamics in optical lattices. _Phys. Rev. A_ 85, 033620 (2012). Article  ADS  Google Scholar  * Dauphin, A. & Goldman, N.

Extracting the Chern number from the dynamics of a Fermi gas: Implementing a quantum Hall bar for cold atoms. _Phys. Rev. Lett._ 111, 135302 (2013). Article  ADS  Google Scholar  *

Aidelsburger, M. et al. Experimental realization of strong effective magnetic fields in an optical lattice. _Phys. Rev. Lett._ 107, 255301 (2011). Article  ADS  Google Scholar  *

Aidelsburger, M. et al. Realization of the Hofstadter Hamiltonian with ultracold atoms in optical lattices. _Phys. Rev. Lett._ 111, 185301 (2013). Article  ADS  Google Scholar  * Atala, M.

et al. Direct measurement of the Zak phase in topological Bloch bands. _Nature Phys._ 9, 795–800 (2013). Article  ADS  Google Scholar  * Abanin, D. A., Kitagawa, T., Bloch, I. & Demler,

E. Interferometric approach to measuring band topology in 2D optical lattices. _Phys. Rev. Lett._ 110, 165304 (2013). Article  ADS  Google Scholar  * LeBlanc, L. J. et al. Observation of a

superfluid Hall effect. _Proc. Natl Acad. Sci. USA_ 109, 10811–10814 (2012). Article  ADS  Google Scholar  * Harper, P. G. Single band motion of conduction electrons in a uniform magnetic

field. _Proc. Phys. Soc. A_ 68, 879–892 (1955). Article  ADS  Google Scholar  * Azbel, M. Y. Energy spectrum of a conduction electron in a magnetic field. _Zh. Eksp. Teor. Fiz._ 46, 929–946

(1964) [_Sov. Phys. JETP_ 19, 634–645 (1964)] Google Scholar  * Jaksch, D. & Zoller, P. Creation of effective magnetic fields in optical lattices: The Hofstadter butterfly for cold

neutral atoms. _New J. Phys._ 5, 56 (2003). Article  ADS  Google Scholar  * Gerbier, F. & Dalibard, J. Gauge fields for ultracold atoms in optical superlattices. _New J. Phys._ 12,

033007 (2010). Article  ADS  Google Scholar  * Mueller, E. J. Artificial electromagnetism for neutral atoms: Escher staircase and Laughlin liquids. _Phys. Rev. A_ 70, 041603 (2004). Article

  ADS  Google Scholar  * Kolovsky, A. R. Creating artificial magnetic fields for cold atoms by photon-assisted tunneling. _Europhys. Lett._ 93, 20003 (2011). Article  ADS  Google Scholar  *

Baur, S. K., Schleier-Smith, M. H. & Cooper, N. R. Dynamic optical superlattices with topological bands. _Phys. Rev. A_ 89, 051605 (2014). Article  ADS  Google Scholar  * Miyake, H.,

Siviloglou, G. A., Kennedy, C. J., Burton, W. C. & Ketterle, W. Realizing the Harper Hamiltonian with laser-assisted tunneling in optical lattices. _Phys. Rev. Lett._ 111, 185302 (2013).

Article  ADS  Google Scholar  * Sørensen, A. S., Demler, E. & Lukin, M. D. Fractional quantum Hall states of atoms in optical lattices. _Phys. Rev. Lett._ 94, 086803 (2005). Article 

ADS  Google Scholar  * Goldman, N. & Dalibard, J. Periodically-driven quantum systems: Effective Hamiltonians and engineered gauge fields. _Phys. Rev. X_ 4, 031027 (2014). Google Scholar

  * Bukov, M., D’Alessio, L. & Polkovnikov, A. Universal high-frequency behavior of periodically driven systems: From dynamical stabilization to Floquet engineering. Preprint at

http://arXiv.org/abs/1407.4803 (2014) * Lignier, H. et al. Dynamical control of matter-wave tunneling in periodic potentials. _Phys. Rev. Lett._ 99, 220403 (2007). Article  ADS  Google

Scholar  * Struck, J. et al. Quantum simulation of frustrated classical magnetism in triangular optical lattices. _Science_ 333, 996–999 (2011). Article  ADS  Google Scholar  * Xiao, D.,

Chang, M-C. & Niu, Q. Berry phase effects on electronic properties. _Rev. Mod. Phys._ 82, 1959–2007 (2010). Article  ADS  MathSciNet  Google Scholar  * Nascimbène, S. et al. Experimental

realization of plaquette resonating valence-bond states with ultracold atoms in optical superlattices. _Phys. Rev. Lett._ 108, 205301 (2012). Article  ADS  Google Scholar  * Jotzu, G. et

al. Experimental realisation of the topological Haldane model. Preprint at http://arXiv.org/abs/1406.7874 (2014) Download references ACKNOWLEDGEMENTS We acknowledge fruitful discussions with

J. Dalibard and also with A. Dauphin, P. Gaspard, F. Gerbier, F. Grusdt, I. Carusotto, T. Ozawa and H. Price. This work was supported by NIM, the EU (UQUAM, SIQS) and EPSRC Grant No.

EP/K030094/1. M.Aidelsburger was further supported by the Deutsche Telekom Stiftung, M.L. by ExQM and N.G. by the Université Libre de Bruxelles and the FRS-FNRS (Belgium). AUTHOR INFORMATION

Author notes * J. T. Barreiro Present address: Present address: Department of Physics, University of California, San Diego, California 92093, USA., AUTHORS AND AFFILIATIONS * Fakultät für

Physik, Ludwig-Maximilians-Universität, Schellingstrasse 4, 80799 München, Germany M. Aidelsburger, M. Lohse, C. Schweizer, M. Atala, J. T. Barreiro & I. Bloch * Max-Planck-Institut für

Quantenoptik, Hans-Kopfermann-Strasse 1, 85748 Garching, Germany M. Aidelsburger, M. Lohse, C. Schweizer, M. Atala, J. T. Barreiro & I. Bloch * Collège de France, 11 place Marcelin

Berthelot & Laboratoire Kastler Brossel, CNRS, UPMC, ENS, 24 rue Lhomond 75005 Paris, France, S. Nascimbène & N. Goldman * T. C. M. Group, Cavendish Laboratory, J.J. Thomson Avenue,

Cambridge CB3 0HE, UK N. R. Cooper * Center for Nonlinear Phenomena and Complex Systems, Université Libre de Bruxelles (U.L.B.), B-1050 Brussels, Belgium N. Goldman Authors * M. Aidelsburger

View author publications You can also search for this author inPubMed Google Scholar * M. Lohse View author publications You can also search for this author inPubMed Google Scholar * C.

Schweizer View author publications You can also search for this author inPubMed Google Scholar * M. Atala View author publications You can also search for this author inPubMed Google Scholar

* J. T. Barreiro View author publications You can also search for this author inPubMed Google Scholar * S. Nascimbène View author publications You can also search for this author inPubMed 

Google Scholar * N. R. Cooper View author publications You can also search for this author inPubMed Google Scholar * I. Bloch View author publications You can also search for this author

inPubMed Google Scholar * N. Goldman View author publications You can also search for this author inPubMed Google Scholar CONTRIBUTIONS M.Aidelsburger, M.L. and C.S. performed the

experiment. All authors contributed to the design of the experiment, the theoretical and data analysis, and to the writing of the paper. I.B. and N.G. supervised the project. CORRESPONDING

AUTHOR Correspondence to M. Aidelsburger. ETHICS DECLARATIONS COMPETING INTERESTS The authors declare no competing financial interests. SUPPLEMENTARY INFORMATION SUPPLEMENTARY INFORMATION

Supplementary Information (PDF 1120 kb) RIGHTS AND PERMISSIONS Reprints and permissions ABOUT THIS ARTICLE CITE THIS ARTICLE Aidelsburger, M., Lohse, M., Schweizer, C. _et al._ Measuring the

Chern number of Hofstadter bands with ultracold bosonic atoms. _Nature Phys_ 11, 162–166 (2015). https://doi.org/10.1038/nphys3171 Download citation * Received: 24 July 2014 * Accepted: 30

October 2014 * Published: 22 December 2014 * Issue Date: February 2015 * DOI: https://doi.org/10.1038/nphys3171 SHARE THIS ARTICLE Anyone you share the following link with will be able to

read this content: Get shareable link Sorry, a shareable link is not currently available for this article. Copy to clipboard Provided by the Springer Nature SharedIt content-sharing

initiative