Reader TTS

ABSTRACT The experimental advances in the study of time-dependent phenomena has opened a new path to investigating the complex electronic structure of strongly correlated and topological

materials. Yu-Shiba-Rusinov (YSR) states induced by magnetic impurities in _s_-wave superconductors provide an ideal candidate system to study the response of a system to time-dependent

manipulations of the magnetic environment. Here, we show that by imposing a time-dependent change in the magnetic exchange coupling, by changing the relative alignment of magnetic moments in

an impurity dimer, or through a periodic drive of the impurity moment, one can tune the system through a time-dependent quantum phase transition, in which the system undergoes a transition

from a singlet to a doublet ground state. We show that the electronic response of the system to external perturbations can be imaged through the time-dependent differential conductance,

_d__I_(_t_)/_d__V_, which, in analogy to the equilibrium case, is proportional to a non-equilibrium local density of states. Our results open the path to visualizing the response of complex

quantum systems to time-dependent external perturbations. SIMILAR CONTENT BEING VIEWED BY OTHERS FIELD-INDUCED LIFSHITZ TRANSITION IN THE MAGNETIC WEYL SEMIMETAL CANDIDATE PRALSI Article

Open access 10 January 2023 THE DISCOVERY OF THREE-DIMENSIONAL VAN HOVE SINGULARITY Article Open access 14 March 2024 NONEQUILIBRIUM CHARGE-DENSITY-WAVE ORDER BEYOND THE THERMAL LIMIT

Article Open access 03 May 2021 INTRODUCTION The experimental ability to probe and manipulate complex electronic correlations at the femtosecond time scale has opened unprecedented

opportunities for the study of non-equilibrium quantum phenomena in strongly correlated or topological materials1,2,3,4,5. The development of next-generation spintronics and quantum

computing applications requires the control of magnetic environments not only on similar time scales, but also on nanoscopic length scales. The latter has been achieved by using scanning

tunneling spectroscopy (STS) techniques that have enabled the writing of magnetic skyrmions6, the tuning of local magnetic exchange couplings7,8,9, and, in combination with electron spin

resonance (ESR) techniques, the rotation of individual magnetic moments in impurity clusters10. These techniques were successfully applied9 to tune the energy of Yu–Shiba–Rusinov (YSR)

states11,12,13—induced by magnetic impurities placed on the surface of an _s_-wave superconductor—and thus to drive the system through a quantum phase transition14,15,16,17. While the

required control of magnetic environments on electronic time scales has yet to be achieved, recent progress10 has raised the question not only of how quantum phenomena—such as quantum phase

transitions—can be manipulated on the (electronic) femtosecond or picosecond time scale, but also of how such time-dependent phenomena can be described theoretically and visualized

experimentally. In this article, we address this question by investigating the manipulation of YSR states11,12,13 on electronic time scales. By using two different theoretical methods based

on the non-equilibrium Keldysh formalism18,19,20 we study the non-equilibrium emergence and manipulation of YSR states in response to external perturbations of the magnetic environment. We

show that the time evolution of YSR states can be visualized through the time-dependent differential conductance, _d__I_(_t_)/_d__V_, which, in analogy to the equilibrium case, is

proportional to a non-equilibrium local density of states (LDOS), _N_neq. These findings allow us to study the time-dependent phase transition of the system from a singlet to a doublet

ground state by subjecting it to a time-dependent change in either the strength of a magnetic impurity’s exchange coupling, or in the relative orientation of moments in a magnetic dimer, or

by externally driving a periodic precession of an impurity’s magnetic moment. Moreover, we show that the extent to which the system is driven out-of-equilibrium is controlled by the time

scale over which perturbations occur, and is directly reflected in the time and frequency dependence of _N_neq. Finally, our formalism reveals the transient behavior between static and

periodically driven magnetic structures, allowing us to visualize the emergence of Floquet YSR states21,22,23. Our work thus provides a theoretical framework to study the emergence of

non-equilibrium phenomena on electronic time and nanoscopic length scales in complex materials. RESULTS THEORETICAL METHOD To study the non-equilibrium emergence and manipulation of YSR

states, we consider magnetic impurities that are placed on the surface of an _s_-wave superconductor, with the exchange coupling or orientation of magnetic moments being time-dependent. Such

a system is described by the Hamiltonian \({{{{{{{\mathcal{H}}}}}}}}={{{{{{{{\mathcal{H}}}}}}}}}_{0}+{{{{{{{\mathcal{U}}}}}}}}(t)\), where $${{{{{{{{\mathcal{H}}}}}}}}}_{0} =

-{t}_{{{{{{{{\rm{e}}}}}}}}}\mathop{\sum}\limits_{\langle {{{{{{{\bf{r}}}}}}}},{{{{{{{{\bf{r}}}}}}}}}^{\prime}\rangle ,\sigma }{c}_{{{{{{{{\bf{r}}}}}}}},\sigma }^{{{{\dagger}}}

}{c}_{{{{{{{{{\bf{r}}}}}}}}}^{\prime},\sigma }-\mu \mathop{\sum}\limits_{{{{{{{{\bf{r}}}}}}}}}{c}_{{{{{{{{\bf{r}}}}}}}},\sigma }^{{{{\dagger}}} }{c}_{{{{{{{{\bf{r}}}}}}}},\sigma }\\ \quad

+{\Delta }_{0}\mathop{\sum}\limits_{{{{{{{{\bf{r}}}}}}}}}\left({c}_{{{{{{{{\bf{r}}}}}}}},\uparrow }^{{{{\dagger}}} }{c}_{{{{{{{{\bf{r}}}}}}}},\downarrow }^{{{{\dagger}}} }+h.c.\right),\\

{{{{{{{\mathcal{U}}}}}}}}(t) = \,J(t)\mathop{\sum}\limits_{{{{{{{{\bf{R}}}}}}}},\alpha ,\beta }{c}_{{{{{{{{\bf{R}}}}}}}},\alpha }^{{{{\dagger}}}

}{\left[{{{{{{{{\bf{S}}}}}}}}}_{{{{{{{{\bf{R}}}}}}}}}(t)\cdot {{{{{{{\boldsymbol{\sigma }}}}}}}}\right]}_{\alpha \beta }{c}_{{{{{{{{\bf{R}}}}}}}},\beta },$$ (1) where −_t_e is the

nearest-neighbor hopping parameter on a square lattice, _μ_ is the chemical potential, Δ0 is the superconducting _s_-wave order parameter, and \({c}_{{{{{{{{\bf{r,\sigma

}}}}}}}}}^{{{{\dagger}}} }\) creates an electron with spin _σ_ at site R. _J_ is the magnetic exchange coupling and SR is the impurity spin at site R with the last sum running over all

impurity positions. Since the hard superconducting _s_-wave gap suppresses Kondo screening17,24, we take the magnetic impurity spin as classical in nature17, i.e.,

\({{{{{{{{\bf{S}}}}}}}}}_{{{{{{{{\bf{r}}}}}}}}}=S(\sin \theta \cos \phi ,\sin \theta \sin \phi ,\cos \theta )\), where _θ_ and _ϕ_ are the polar and azimuthal angles, respectively. Finally,

we note that the perturbation \({{{{{{{\mathcal{U}}}}}}}}(t)\) is purely magnetic in nature, and thus does not break particle–hole symmetry. To theoretically study the electronic response of

the system to a time-dependent _J_ or SR, we recall that the primary experimental probe in the study of YSR states is the differential conductance, _d__I_/_d__V_, measured in STS

experiments17. We therefore compute the time-dependent current flowing between an STS tip and the superconductor using a formulation of the non-equilibrium Keldysh formalism in the

interaction representation18,19,25, yielding $$I(V,t)=\frac{e}{\hslash }(-{t}_{{{{{{{{\rm{tun}}}}}}}}})\mathop{\sum}\limits_{\sigma }\left[{G}_{ts}^{{ < }}(\sigma ,t,t)-{G}_{st}^{{ <

}}(\sigma ,t,t)\right],$$ (2) where _t_tun is the tunneling amplitude between the tip and the superconductor, _V_ is the applied bias, and \({G}_{ts}^{\,{ < }}\) is the spin-dependent,

equal-time lesser Green’s function between the tip (_t_) and the site in the superconductor (_s_) that the electrons tunnel into25. Due to the picometer spatial resolution achieved in STS

experiments26, we here assume that the electrons tunnel into a single site of the superconductor only. To compute \({G}_{ts}^{\,{ < }}\), we employ the Keldysh Dyson equations in real

time, given by $${\bar{G}}_{ts}^{\,{ < }}(t,t) = \,{\bar{g}}_{ts}^{\,{ < }}(t,t)+\int

\,d{t}_{1}{\bar{g}}_{ts}^{{{{{{{{\rm{r}}}}}}}}}(t,{t}_{1})\bar{{{{{{{{\mathcal{U}}}}}}}}}({t}_{1}){\bar{G}}_{ss}^{\,{ < }}({t}_{1},t)\\ \quad +\int \,d{t}_{1}{\bar{g}}_{ts}^{\,{ <

}}(t,{t}_{1})\bar{{{{{{{{\mathcal{U}}}}}}}}}({t}_{1}){\bar{G}}_{ss}^{{{{{{{{\rm{a}}}}}}}}}({t}_{1},t)\\ {\bar{G}}_{ts}^{{{{{{{{\rm{a}}}}}}}}}({t}^{\prime},t )=

\,{\bar{g}}_{ts}^{{{{{{{{\rm{a}}}}}}}}}({t}^{\prime},t)+\int

\,d{t}_{1}{\bar{g}}_{ts}^{{{{{{{{\rm{a}}}}}}}}}({t}^{\prime},{t}_{1})\bar{{{{{{{{\mathcal{U}}}}}}}}}({t}_{1}){\bar{G}}_{ss}^{{{{{{{{\rm{a}}}}}}}}}({t}_{1},t),$$ (3) where \({\bar{g}}^{\,{

< }\,,r,a}\) are the equilibrium lesser, retarded and advanced Greens function matrices of the unperturbed system in Nambu space, and \(\bar{{{{{{{{\mathcal{U}}}}}}}}}(t)\) is the

time-dependent perturbation matrix arising from Eq. (1). By discretizing time, Eq. (3) transforms into a set of coupled matrix equations, allowing us to obtain a closed form for

\({G}_{ts}^{\,{ < }}(t,t)\) (for details, see Supplementary Note 1). Below all times are given in units of _τ_e = ℏ/_t_e which implies that for typical values of _t_e of a few hundred

meV, _τ_e is of the order of a few femtoseconds. As the calculation of _d__I_/_d__V_ using Eq. (2) is computationally intensive, we also employ an alternative, computationally more efficient

approach using the Heisenberg representation of the Keldysh formalism19,20. This approach utilizes the fact that in equilibrium, the differential conductance is proportional to the local

density of states \(N({{{{{{{\bf{r}}}}}}}},\omega )=-{{{{{{{\rm{Im}}}}}}}}\,{g}^{{{{{{{{\rm{r}}}}}}}}}({{{{{{{\bf{r,\; r}}}}}}}},\omega )/\pi\). To define an analogous quantity

out-of-equilibrium, we note that in the presence of a time-dependent perturbation, \({G}^{{{{{{{{\rm{r}}}}}}}}}(t,{t}^{\prime})\) depends explicitly on _t_ and \({t}^{\prime}\). Setting

\({t}^{\prime}=t-\Delta t\) and performing a Fourier transformation with regards to Δ_t_, we obtain a time and frequency dependent Greens function _G_r(_t_, _ω_), allowing us to define a

"non-equilibrium” density of states, _N_neq, via $${N}_{{{{{{{{\rm{neq}}}}}}}}}({{{{{{{\bf{r}}}}}}}},\sigma ,t,\omega )=-\frac{1}{\pi

}{{{{{{{\rm{Im}}}}}}}}{G}^{{{{{{{{\rm{r}}}}}}}}}({{{{{{{\bf{r}}}}}}}},{{{{{{{\bf{r}}}}}}}},\sigma ,t,\omega ),$$ (4) where _G_r(_t_, _ω_) is obtained from

$$\left[{{{{{{{\rm{i}}}}}}}}\frac{d}{dt}+\omega +i\Gamma -\hat{H}(t)\right]{\hat{G}}^{{{{{{{{\rm{r}}}}}}}}}\left(t,\omega \right)=\hat{1},$$ (5) for an arbitrary time dependence of the

Hamiltonian. Here, \(\hat{H}(t)\), \({\hat{G}}^{{{{{{{{\rm{r}}}}}}}}}(t,\omega )\) and \(\hat{1}\) are matrices in real and Nambu space (for details, see Supplementary Note 2), and _τ_Γ = 

ℏ/Γ is the quasi-particle lifetime17. We demonstrate below that _N_neq is proportional to the time-dependent _d__I_(_V_, _t_)/_d__V_, and thus represents a physical observable that describes

the non-equilibrium time evolution of the superconductor’s electronic structure. NON-EQUILIBRIUM EMERGENCE OF YSR STATES We begin by considering the question of how a YSR state emerges when

a single magnetic impurity is placed on the surface of an _s_-wave superconductor at time _t_ = 0. To this end, we consider a perturbation of the form $${{{{{{{\mathcal{U}}}}}}}}(t\ge

0)=JS\mathop{\sum}\limits_{\alpha ,\beta }{c}_{{{{{{{{\bf{r}}}}}}}},\alpha }^{{{{\dagger}}} }{\sigma }_{\alpha \beta }^{z}{c}_{{{{{{{{\bf{r}}}}}}}},\beta },$$ (6) where _S_ is the magnitude

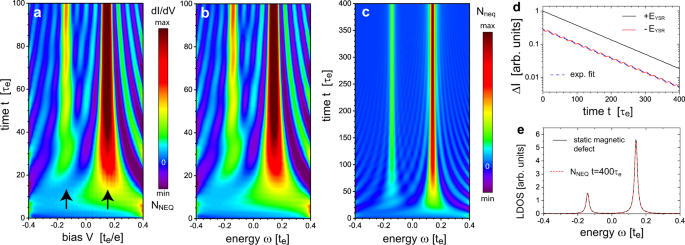
of the impurity spin, and \({{{{{{{\mathcal{U}}}}}}}}(t\, < \,0)=0\). In Fig. 1a, we present the resulting time and bias dependence of _d__I_(_V_, _t_)/_d__V_ as obtained from Eq. (2)

(due to the significant computational resources required, the calculation of _d__I_/_d__V_ is limited to _t_ ≤ 100_τ_e). At _t_ = 0, the LDOS is that of an unperturbed _s_-wave

superconductor without a magnetic impurity, exhibiting a hard gap and coherence peaks at ± Δ0. As the magnetic impurity is placed on the surface, spectroscopic weight from the gap edges

begins to be transferred into the gap, creating at first a broad peak centered around the energy of the emerging YSR state (see black arrows in Fig. 1a). This shift of spectroscopic weight

occurs in a wave-like form, as reflected in the stripe-like patterns of _d__I_(_V_, _t_)/_d__V_ in Fig. 1a27,28. With increasing time, the width of the peaks decreases, while their height

increases, which is a direct consequence of the uncertainty principle: _t_Δ_E_ ≥ ℏ implies that as the time over which the impurity is located on the surface increases, the YSR state’s

energy uncertainty, i.e., the peaks’ width, decreases. To elucidate the relation between _d__I_/_d__V_ and _N_neq, we note that for the perturbation of Eq. (6), the solution of Eq. (5) is

given by $${\hat{G}}^{{{{{{{{\rm{r}}}}}}}}}(t,\omega ) = \left[1-{e}^{{{{{{{{\rm{i}}}}}}}}\left(\omega \hat{1}-{\hat{H}}^{+}\right)t}{e}^{-\Gamma

t}\right]{\hat{g}}_{+}^{{{{{{{{\rm{r}}}}}}}}}(\omega )\\ \quad +{e}^{{{{{{{{\rm{i}}}}}}}}\left(\omega \hat{1}-{\hat{H}}^{+}\right)t}{e}^{-\Gamma t}{\hat{g}}_{0}^{{{{{{{{\rm{r}}}}}}}}}(\omega

)$$ (7) where \({\hat{g}}_{0,+}^{{{{{{{{\rm{r}}}}}}}}}\) are the equilibrium Green’s functions for a system without and with an impurity, respectively, and \({\hat{H}}^{+}\) is the full

Hamiltonian for _t_ ≥ 0. We thus have \({\hat{G}}^{{{{{{{{\rm{r}}}}}}}}}(t=0,\omega )={\hat{g}}_{0}^{{{{{{{{\rm{r}}}}}}}}}(\omega )\) and \({\hat{G}}^{{{{{{{{\rm{r}}}}}}}}}(t\to \infty

,\omega )={\hat{g}}_{+}^{{{{{{{{\rm{r}}}}}}}}}(\omega )\). A comparison of _N_neq obtained from Eqs. (4) and (7) (see Fig. 1b) with _d__I_/_d__V_ (see Fig. 1a) shows remarkable quantitative

agreement (up to an overall scaling factor) in the (_ω_, _t_)-plane (a more detailed comparison is provided in Supplementary Movie 1). This suggests that _d__I_(_V_, _t_)/_d__V_ ~ 

_N_neq(_t_, _ω_ = _e__V_), implying that _N_neq represents a physical observable that describes the non-equilibrium time evolution of the superconductor’s electronic structure. Since _N_neq

is computed in the absence of a tip, we expect _d__I_/_d__V_ ~ _N_neq to hold only in the weak-tunneling regime, similar to the equilibrium case17. As the calculation of _N_neq is

computationally less demanding than that of _d__I_/_d__V_, we can now study the time-evolution of the system up to much larger times using _N_neq, as shown in Fig. 1c, where we present

_N_neq(_t_, _ω_) up to _t_ = 400_τ_e. Eq. (7) suggests that when _ω_ = ± _E_YSR, with _E_YSR being the YSR state energy, the oscillatory term, \(\sim {e}^{i\left(\omega

\hat{1}-\hat{{H}^{+}}\right)t}\) is identical to one, and _N_neq relaxes exponentially to the static case for _t_ → _∞_, with the relaxation time given by _τ_Γ28, which is identical to the

lifetime of the YSR state. This is confirmed by a log-plot of the deviation of the YSR peaks’ height from the equilibrium value, Δ_I_, as obtained from _N_neq in Fig. 1C, as a function of

time presented in Fig. 1d, where the dashed line corresponds to ~ _e_−Γ_t_/ℏ. Finally, a comparison of the equilibrium LDOS in the presence of a static magnetic impurity with _N_neq(_t_, 

_ω_) at _t_ = 400_τ_e (see Fig. 1e) shows very good agreement, demonstrating that this formalism allows us to study the system during the entire equilibration process. We note that the

agreement between _d__I_/_d__V_ and _N_neq is independent of the specific value of Γ chosen (see Supplementary Movie 2), as Γ only controls the relaxation time of the process. Moreover, for

more realistic parameters sets, the relaxational dynamics discussed above can be observed on time scales up to 100 ps (see Supplementary Fig. 1). DYNAMICAL TUNING OF QUANTUM PHASE

TRANSITIONS An intriguing phenomenon associated with the presence of a magnetic impurity is the possibility to drive the superconductor through a quantum phase transition between a singlet

and doublet ground state by either tuning _J_ through a critical value, \({J}_{{{{{{{{\rm{c}}}}}}}}}={(\pi {N}_{0}S)}^{-1}\),14,15,16,17, where _N_0 is the normal state density of states at

the Fermi energy, or by changing the distance or relative spin alignment of two or more impurities29,30. This phase transition is accompanied by a zero-energy crossing of the YSR peaks in

the LDOS. To investigate whether such a phase transition can also be induced using non-equilibrium techniques31,32, we begin by considering the case of a time-dependent exchange coupling

_J_, which is motivated by the experimental ability to significantly vary _J_ using STS techniques7,8,9. Specifically, we increase the exchange coupling from an initial value _J_i < _J_c

at _t_ = 0 to a final value _J_f > _J_c at a time _t_ = Δ_t_, using the time evolution \(J(t)={J}_{{{{{{{{\rm{i}}}}}}}}}+({J}_{{{{{{{{\rm{f}}}}}}}}}-{J}_{{{{{{{{\rm{i}}}}}}}}}){\sin

}^{2}\left(\frac{\pi t}{2\Delta t}\right)\) for 0 ≤ _t_ ≤ Δ_t_ as shown in Fig. 2a. A plot of the equilibrium LDOS for _J_ = _J__i_,_f_ in Fig. 2b demonstrates that the zero energy crossing

of the YSR peaks at the phase transition exchanges the spin projection of their particle- and hole-like branches14,15,16,17, which can be mapped using spin-polarized STS33. The time

evolution of the phase transition can thus be best visualized by considering the spin-resolved _N_neq(_σ_, _t_, _ω_), shown in Fig. 2c, d for _σ_ =  _↑_ and _σ_ =  _↓_, respectively. While

the change in _J_ over a finite time Δ_t_ leads to significant oscillations in _N_neq, a zero-energy crossing of the YSR peaks, indicating a time-dependent phase transition14,15,16,17, can

still be identified. Moreover, _N_neq reveals several noteworthy features. First, the spectral weight of the YSR peaks is decreased during the zero-energy crossing, concomitant with the

phase transition. Second, a substantial redistribution of the YSR state’s spectral weight begins to occur only after _t_ ≈ 50_τ_e = Δ_t_/2, indicating a delayed response of the system to the

imposed change in _J_. This is particularly evident from comparison of the time-dependent YSR peak height at the final energy position in Fig. 2e with _J_(_t_) shown in Fig. 2a. Third,

substantial oscillations in _N_neq at the energy of the original YSR peaks persist up to time scales significantly longer than Δ_t_, and the spectral weight of these oscillations is shifted

to the final energy position of the YSR states in a wave-like pattern, as evidenced by the stripe-like pattern in _N_neq (see Fig. 2c, d). Finally, a comparison of _d__I_/_d__V_ and _N_neq

shown in Supplementary Movie 3 again reveals good quantitative agreement between them, further supporting our conclusion that _d__I_/_d__V_ ~ _N_neq. TWISTING A SPIN DIMER The superconductor

can also be tuned through a quantum phase transition by changing the relative alignment of the spins of two impurities in close proximity from antiparallel to parallel29, even if each of

the two impurities possesses an exchange coupling smaller than the critical value, _J_c. Such a change in the spins’ relative alignment in an impurity dimer has been achieved using ESR

techniques10. For antiparallel alignment, the YSR states associated with each impurity cannot hybridize as the spin quantum number of their particle- and hole-like branches are opposite. The

LDOS thus exhibits two pairs of degenerate YSR states with opposite spin quantum numbers29, as shown in Fig. 3a, b. When the alignment is rotated to parallel, the YSR states hybridize,

leading to an energy splitting between them and the emergence of four peaks in the LDOS (see Fig. 3a, b). Plotting the equilibrium LDOS as a function of the angle _θ_ between the spins (Fig.

 3c, d), corresponding to the adiabatic limit of the rotation, we find that the energy splitting increases with decreasing _θ_, leading to a zero energy crossing of two of the YSR peaks, and

a concomitant phase transition from a singlet to a doublet ground state. The question naturally arises as to the time dependence of such a phase transition when the relative alignment is

changed over a finite time Δ_t_, as was done in ref. 10 through the application of an ESR _π_ pulse. To address this question, we consider for concreteness the rotation of one of the two

impurity spins through the _x__z_ plane described by \({{{{{{{{\bf{S}}}}}}}}}_{{{{{{{{\bf{r}}}}}}}}}=S[\sin \theta (t),0,\cos \theta (t)]\), with the time-dependent polar angle given by

$$\theta (t)=\pi \,{\cos }^{2}\left(\frac{\pi }{2\Delta t}t\right),\quad {{{{{{{\rm{for}}}}}}}}\,0\le t\le \Delta t.$$ (8) In Fig. 3e, f we present the resulting spin-resolved _N_neq(_σ_, 

_t_, _ω_) for Δ_t_ = 200_τ_e, with the spins being antiparallel for _t_ = 0 and parallel for _t_ = Δ_t_. As before, we can identify a time-dependent zero-energy crossing of the YSR peaks

(see yellow arrows in Fig. 3e, f) indicating a phase transition from a singlet to a doublet ground state. We note that the angular dependence of the equilibrium LDOS shown in Fig. 3c, d,

corresponds to the time dependence of _N_neq between 0 ≤ _t_ ≤ Δ_t_ (see white dashed line in Fig. 3e, f) in the adiabatic limit of Δ_t_ → _∞_. A comparison of the equilibrium LDOS with

_N_neq in Fig. 3e, f thus reveals that a finite Δ_t_ leads to significant time-dependent oscillations in _N_neq, in particular near the energy positions of the YSR peaks at _t_ = 0, \(\pm

{E}_{{{{{{{{\rm{YSR}}}}}}}}}^{(0)}\). To investigate how the strength of these oscillations depends on Δ_t_, we plot in Fig. 3g, h\({N}_{{{{{{{{\rm{neq}}}}}}}}}({t}_{\Delta },\pm

{E}_{{{{{{{{\rm{YSR}}}}}}}}}^{(0)})\) as a function of time after the rotation is completed, i.e., _t_Δ = _t_ − Δ_t_, for two different values of Δ_t_. We find that the amplitude of the

oscillations in _N_neq(_t_Δ, _ω_) increases with decreasing Δ_t_ as the system is more strongly driven out of equilibrium. This result is consistent with the expectation that in the

quasi-static, adiabatic limit, Δ_t_ → _∞_, these oscillations vanish, and _N_neq becomes identical to the equilibrium LDOS in Fig. 3c, d, when plotted as a function of angle. EMERGENCE OF

FLOQUET YSR STATES The formalism presented above can also be employed to investigate periodically driven systems, which are typically studied using the Floquet formalism21,22,23, and the

transient behavior of quantum systems between the static and periodically driven limits. The latter is of particular interest since heating effects in interacting systems34 could potentially

destroy novel quantum states, such as Floquet topological insulators22 or Floquet topological superconductors35 even before they are fully formed. To study such transient behavior, we

consider the case of a single magnetic impurity, and study the time evolution from a single static impurity spin at _t_ = 0 to an impurity spin that rotates periodically in the _x__z_-plane

with a driving frequency of _ω_0 = 2_π_/_T_. We note that the periodic rotation of the impurity’s spin leads to a splitting of the (static) YSR peaks in the density of states36, with the

splitting given by _ω_0. This is shown in Fig. 4a where we present the evolution of the Floquet LDOS _N_Fl with increasing driving frequency _ω_0 (for details, see Supplementary Note 3).

Since, _J_ = 2.8_t_e > _J_c, and since for _ω_ → _∞_ the YSR states merge with the continuum, as the scattered electrons see a vanishing effective _J_, a zero-energy crossing of the YSR

peaks in _N_Fl occurs at some critical value of the driving frequency, _ω__c_, indicating a phase transition (see yellow arrow in Fig. 4a) from a doublet to a singlet ground state. To

investigate the transient behavior from a static to a periodically rotating impurity spin, we employ a time-dependent polar angle given by \(\theta (t)={\omega }_{0}t\tanh \left(5{\omega

}_{0}t/\pi \right)\) for 0 ≤ _t_ ≤ _T_/2 and _θ_(_t_) = _ω_0_t_ for _t_ ≥ _T_/2. The resulting _N_neq shown in Fig. 4b for _ω_0 = 0.2_t_e/ℏ clearly reveals the emergence of the YSR peak

splitting and of four YSR peaks with increasing time. Since _ω_0 > _ω__c_ and _J_ > _J_c, we find that with increasing time, there is a substantial transfer of spectral weight across

zero energy, indicating a time-dependent phase transition (see black arrow in Fig. 4b). We note that for _t_ ≫ _T_, the system is described by a Hamiltonian that is periodic in time, such

that _N_neq is expected to coincide with the Floquet LDOS _N_Fl. We find that already for _t_ = 400_τ_e, _N_neq is in very good quantitative agreement with _N_Fl (see Supplementary Fig. 3

and Supplementary Note 4) that supports the validity not only of the non-equilibrium formalism presented above to describe periodically driven systems, but also the definition of _N_neq as

an observable physical quantity. Moreover, we note that while the total, spin-summed _N_neq(_t_, _ω_) does not exhibit any signature of the rotation period (consistent with a

time-independent total _N_Fl), the spin-resolved _N_neq(_σ_, _t_, _ω_) shown in Fig. 4c, d does, an effect refereed to as micromotion37,38. As expected from a rotation of the spin in the

_x__z_-plane, we find that _N_neq for _σ_ = _↑_ and _σ_ = _↓_ are out of phase, i.e., shifted by half a period _T_. A comparison of _d__I_/_d__V_ and _N_neq shown in Supplementary Movie 4

again reveals good quantitative agreement between these quantities, further supporting our conclusion that _d__I_/_d__V_ ~ _N_neq. Due to the numerical complexity in computing _d__I_/_d__V_,

we were restricted to time scales _t_ ≤ 20_τ_e for this comparison. DISCUSSION The ability to understand the non-equilibrium response of complex quantum systems to external perturbations on

electronic (femtosecond) timescales and nanoscopic length scales is crucial not only for advancing our theoretical understanding of these systems, but also for the development of next

generation spintronics and quantum computing applications. Here, we have shown that time-dependent manipulations of magnetic impurities—leading to changes in their exchange coupling or

relative spin alignment—not only allow us to study the emergence of quantum phenomena, such as the formation of YSR states, but can also give rise to new intriguing phenomena, such as a

time-dependent phase transition. Studying and understanding the response of complex quantum system to external perturbations will be crucial for realizing topological quantum gates, and the

required braiding of Majorana fermions. Indeed, the formalism we have presented here provides a suitable approach to study the non-equilibrium response of topological superconductors, and in

particular Majorana modes39,40,41. One could envision a few different approaches to realizing experiments capable of probing the relaxational dynamics of the quantum phenomena described

above. To this end, experiments would have to be performed on the scale of the lifetime _τ_Γ of YSR states, which for more realistic parameters (see Supplementary Fig. 1) might extend up to

100ps. For example, ESR/STS techniques could in general be used to rotate magnetic moments (see ref. 10, which is relevant for the case discussed in Fig. 2 of our manuscript) or change the

magnetic exchange interaction (see refs. 7,8,9, relevant for the case of Fig. 3) while simultaneously measuring the differential conductance _d__I_/_d__V_. The time scale for spin-flip

processes in ESR/STS experiments is currently on the order of 20 ns10, but could plausibly be further reduced by factors of 10–100 by increasing local magnetic fields, for example, created

by additional magnetic impurities (Lutz, C. P., private communication). Moreover, the quantum state of individual molecules located in a scanning tunneling microscope (STM) cavity can be

manipulated using THz irradiation, with the STM measuring the resulting changes using inelastic tunneling spectroscopy42. Similarly, it might be possible to use light-spin interactions to

induce spin-oscillations on the ps time scale43 in magnetic atoms located in an STM cavity. Which of these possible experimental approaches will ultimately be successful can only be

determined by future work. Finally, we note that in interacting systems (in contrast to the non-interacting system considered here) non-equilibrium perturbations can lead to detrimental

heating effects, which are likely more relevant for periodic drives, such as the one considered in Fig. 4, than for the cases discussed in Figs. 1–3. However, it was shown that even for

periodic drives, there exists a pre-thermalization regime44,45 in which non-equilibrium quantum states can be formed before they are destroyed by heating effects at larger time scales34. How

heating effects and the pre-thermalization regime can be quantum engineered46 in the context of YSR states is an interesting question for future work. METHODS In order to obtain the

differential conductance, we employ Eq. (2) to calculate the current between the tip and the system for a given set of bias voltages and numerically take the derivative with respect to the

bias voltage. The details regarding the calculation of the Green’s functions using the Keldysh formalism can be found in Supplementary Note 1. To calculate the non-equilibrium local density

of states, we employ Eqs. (4) and (5). The local density of states in the static case is computed by representing the Hamiltonian of Eq. (1) as a matrix in real and Nambu space and using

Supplementary Eq. (S31) to calculate the retarded Green’s function matrix \({\hat{g}}^{r}\), from which the LDOS is obtained as \(N({{{{{{{\bf{r}}}}}}}},\sigma ,\omega

)=-{{{{{{{\rm{Im}}}}}}}}\left[{\hat{g}}^{r}({{{{{{{\bf{r}}}}}}}},\sigma ;{{{{{{{\bf{r}}}}}}}},\sigma ,\omega )\right]/\pi\). The Floquet LDOS _N_Fl is calculated using the Floquet formalism,

which is described in Supplementary Note 3. DATA AVAILABILITY Original data are available at https://doi.org/10.5281/zenodo.7116734. CODE AVAILABILITY The codes that were employed in this

study are available from the authors on reasonable request. REFERENCES * Perfetti, L. et al. Time evolution of the electronic structure of 1T - TaS_2 through the insulator-metal transition.

_Phys. Rev. Lett._ 97, 067402 (2006). Article  ADS  Google Scholar  * Schmitt, F. et al. Transient electronic structure and melting of a charge density wave in TbTe3. _Science_ 321,

1649–1652 (2008). Article  ADS  Google Scholar  * Fausti, D. et al. Light-induced superconductivity in a stripe-ordered cuprate. _Science_ 331, 189–191 (2011). Article  ADS  Google Scholar 

* Sobota, J. A. et al. Ultrafast optical excitation of a persistent surface-state population in the topological insulator Bi2Se3. _Phys. Rev. Lett._ 108, 117403 (2012). Article  ADS  Google

Scholar  * McIver, J. W. et al. Light-induced anomalous Hall effect in graphene. _Nat. Phys._ 16, 38–41 (2020). Article  Google Scholar  * Romming, N. et al. Writing and deleting single

magnetic skyrmions. _Science_ 341, 636–639 (2013). Article  ADS  Google Scholar  * Farinacci, L. et al. Tuning the coupling of an individual magnetic impurity to a superconductor: quantum

phase transition and transport. _Phys. Rev. Lett._ 121, 196803 (2018). Article  ADS  Google Scholar  * Yang, K. et al. Tuning the exchange bias on a single atom from 1 mT to 10 T. _Phys.

Rev. Lett._ 122, 227203 (2019). Article  ADS  Google Scholar  * Karan, S. et al. Superconducting quantum interference at the atomic scale. _Nat. Phys._ 18, 893–898 (2022). * Yang, K. et al.

Coherent spin manipulation of individual atoms on a surface. _Science_ 366, 509–512 (2019). Article  ADS  Google Scholar  * Yu, L. Bound state in superconductors with paramagnetic

impurities. _Acta Phys. Sin._ 21, 75–91 (1965). Article  Google Scholar  * Shiba, H. Classical spins in superconductors. _Prog. Theor. Phys._ 40, 435–451 (1968). Article  ADS  Google Scholar

  * Rusinov, A. I. On the theory of gapless superconductivity in alloys containing paramagnetic impurities. _J. Exp. Theor. Phys._ 29, 1101–1106 (1969). ADS  Google Scholar  * Sakurai, A.

Comments on superconductors with magnetic impurities. _Prog. Theor. Phys._ 44, 1472–1476 (1970). Article  ADS  Google Scholar  * Salkola, M. I., Balatsky, A. V. & Schrieffer, J. R.

Spectral properties of quasiparticle excitations induced by magnetic moments in superconductors. _Phys. Rev. B_ 55, 12648 (1997). Article  ADS  Google Scholar  * Bazaliy, Y. B. & Jones,

B. A. Magnetic impurity in a superconductor: local phase transitions and finite size effects. _J. Appl. Phys._ 87, 5561–5563 (2000). Article  ADS  Google Scholar  * Balatsky, A. V., Vekhter,

I. & Zhu, J.-X. Impurity-induced states in conventional and unconventional superconductors. _Rev. Mod. Phys._ 78, 373–433 (2006). Article  ADS  Google Scholar  * Keldysh, L. V. Diagram

technique for non-equilibrium processes. _Soviet Phys. Jetp_ 20, 1018–1026 (1965). * Rammer, J. & Smith, H. Quantum field-theoretical methods in transport theory of metals. _Rev. Mod.

Phys._ 58, 323–359 (1986). Article  ADS  Google Scholar  * Kamenev, A. & Levchenko, A. Keldysh technique and non-linear _σ_-model: Basic principles and applications. _Adv. Phys._ 58,

197–319 (2009). Article  ADS  Google Scholar  * Kohler, S., Lehmann, J. & Hänggi, P. Driven quantum transport on the nanoscale. _Phys. Rep._ 406, 379–443 (2005). Article  ADS  Google

Scholar  * Cayssol, J., Dóra, B., Simon, F. & Moessner, R. Floquet topological insulators. _Phys. Status Solidi RRL_ 7, 101–108 (2013). Article  Google Scholar  * Oka, T. & Kitamura,

S. Floquet engineering of quantum materials. _Annu. Rev. Condens. Matter Phys._ 10, 387–408 (2019). Article  ADS  Google Scholar  * Heinrich, B. W., Pascual, J. I. & Franke, K. J.

Single magnetic adsorbates on s -wave superconductors. _Prog. Surf. Sci._ 93, 1–19 (2018). Article  ADS  Google Scholar  * Caroli, C., Combescot, R., Nozieres, P. & Saint-James, D.

Direct calculation of the tunneling current. _J. Phys. C Solid State Phys._ 4, 916–929 (1971). Article  ADS  Google Scholar  * Hamidian, M. H. et al. Picometer registration of zinc impurity

states in Bi2Sr2CaCu2O8+ _δ_ for phase determination in intra-unit-cell Fourier transform STM. _New J. Phys._ 14, 053017 (2012). Article  ADS  Google Scholar  * Souto, R. S., Martín-Rodero,

A. & Yeyati, A. L. Andreev bound states formation and quasiparticle trapping in quench dynamics revealed by time-dependent counting statistics. _Phys. Rev. Lett._ 117, 267701 (2016).

Article  ADS  Google Scholar  * Taranko, R. & Domański, T. Buildup and transient oscillations of Andreev quasiparticles. _Phys. Rev. B_ 98, 075420 (2018). Article  ADS  Google Scholar  *

Morr, D. K. & Stavropoulos, N. A. Quantum interference between impurities: creating novel many-body states in _s_ -wave superconductors. _Phys. Rev. B_ 67, 020502 (2003). Article  ADS 

Google Scholar  * Morr, D. K. & Yoon, J. Impurities, quantum interference, and quantum phase transitions in s -wave superconductors. _Phys. Rev. B_ 73, 224511 (2006). Article  ADS 

Google Scholar  * Seoane Souto, R., Feiguin, A. E., Martín-Rodero, A. & Yeyati, A. L. Transient dynamics of a magnetic impurity coupled to superconducting electrodes: exact numerics

versus perturbation theory. _Phys. Rev. B_ 104, 214506 (2021). Article  ADS  Google Scholar  * Wrześniewski, K., Weymann, I., Sedlmayr, N. & Domański, T. Dynamical quantum phase

transitions in a mesoscopic superconducting system. _Phys. Rev. B_ 105, 094514 (2022). Article  ADS  Google Scholar  * Cornils, L. et al. Spin-resolved spectroscopy of the Yu-Shiba-Rusinov

states of individual atoms. _Phys. Rev. Lett._ 119, 197002 (2017). Article  ADS  Google Scholar  * Weidinger, S. A. & Knap, M. Floquet prethermalization and regimes of heating in a

periodically driven, interacting quantum system. _Sci. Rep._ 7, 45382 (2017). Article  ADS  Google Scholar  * Jiang, L. et al. Majorana fermions in equilibrium and in driven cold-atom

quantum wires. _Phys. Rev. Lett._ 106, 220402 (2011). Article  ADS  Google Scholar  * Kaladzhyan, V., Hoffman, S. & Trif, M. Dynamical Shiba states from precessing magnetic moments in an

s -wave superconductor. _Phys. Rev. B_ 95, 195403 (2017). Article  ADS  Google Scholar  * Goldman, N. & Dalibard, J. Periodically driven quantum systems: effective Hamiltonians and

engineered gauge fields. _Phys. Rev. X_ 4, 031027 (2014). Google Scholar  * Desbuquois, R. et al. Controlling the Floquet state population and observing micromotion in a periodically driven

two-body quantum system. _Phys. Rev. A_ 96, 053602 (2017). Article  ADS  Google Scholar  * Tuovinen, R., Perfetto, E., van Leeuwen, R., Stefanucci, G. & Sentef, M. A. Distinguishing

Majorana zero modes from impurity states through time-resolved transport. _New J. Phys._ 21, 103038 (2019). Article  ADS  MathSciNet  Google Scholar  * Väyrynen, J. I., Pikulin, D. I. &

Lutchyn, R. M. Majorana signatures in charge transport through a topological superconducting double-island system. _Phys. Rev. B_ 103, 205427 (2021). Article  ADS  Google Scholar  *

Barański, J., Barańska, M., Zienkiewicz, T., Taranko, R. & Domański, T. Dynamical leakage of Majorana mode into side-attached quantum dot. _Phys. Rev. B_ 103, 235416 (2021). Article  ADS

  Google Scholar  * Wang, L., Xia, Y. & Ho, W. Atomic-scale quantum sensing based on the ultrafast coherence of an H2 molecule in an STM cavity. _Science_ 376, 401–405 (2022). Article 

ADS  Google Scholar  * Kanda, N. et al. The vectorial control of magnetization by light. _Nat Commun_ 2, 362 (2011). Article  ADS  Google Scholar  * Peng, P., Yin, C., Huang, X., Ramanathan,

C. & Cappellaro, P. Floquet prethermalization in dipolar spin chains. _Nat. Phys._ 17, 444–447 (2021). Article  Google Scholar  * Beatrez, W. et al. Floquet prethermalization with

lifetime exceeding 90 s in a bulk hyperpolarized solid. _Phys. Rev. Lett._ 127, 170603 (2021). Article  ADS  Google Scholar  * Shkedrov, C. et al. Absence of heating in a uniform Fermi gas

created by periodic driving. _Phys. Rev. X_ 12, 011041 (2022). Google Scholar  Download references ACKNOWLEDGEMENTS The authors would like to thank G. Czap, K.J. Franke, C.P. Lutz, H. Kim,

S. Rachel, J. Wiebe, and R. Wiesendanger for stimulating discussions. This work was supported by the U. S. Department of Energy, Office of Science, Basic Energy Sciences, under Award No.

DE-FG02-05ER46225. AUTHOR INFORMATION Author notes * Eric Mascot Present address: School of Physics, University of Melbourne, Parkville, VIC, 3010, Australia AUTHORS AND AFFILIATIONS *

Department of Physics, University of Illinois at Chicago, Chicago, IL, 60607, USA Jasmin Bedow, Eric Mascot & Dirk K. Morr Authors * Jasmin Bedow View author publications You can also

search for this author inPubMed Google Scholar * Eric Mascot View author publications You can also search for this author inPubMed Google Scholar * Dirk K. Morr View author publications You

can also search for this author inPubMed Google Scholar CONTRIBUTIONS D.K.M. conceived and supervised the project. J.B. and E.M. performed the theoretical calculations. D.K.M. wrote the

manuscript, with contributions from all authors. CORRESPONDING AUTHOR Correspondence to Dirk K. Morr. ETHICS DECLARATIONS COMPETING INTERESTS The authors declare no competing interests. PEER

REVIEW PEER REVIEW INFORMATION _Communications Physics_ thanks the anonymous reviewers for their contribution to the peer review of this work. Peer reviewer reports are available.

ADDITIONAL INFORMATION PUBLISHER’S NOTE Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations. SUPPLEMENTARY INFORMATION

MORR_PR FILE SUPPLEMENTARY INFORMATION DESCRIPTION OF ADDITIONAL SUPPLEMENTARY FILES SUPPLEMENTARY MOVIE 1 SUPPLEMENTARY MOVIE 2 SUPPLEMENTARY MOVIE 3 SUPPLEMENTARY MOVIE 4 RIGHTS AND

PERMISSIONS OPEN ACCESS This article is licensed under a Creative Commons Attribution 4.0 International License, which permits use, sharing, adaptation, distribution and reproduction in any

medium or format, as long as you give appropriate credit to the original author(s) and the source, provide a link to the Creative Commons license, and indicate if changes were made. The

images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in a credit line to the material. If material is not

included in the article’s Creative Commons license and your intended use is not permitted by statutory regulation or exceeds the permitted use, you will need to obtain permission directly

from the copyright holder. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/. Reprints and permissions ABOUT THIS ARTICLE CITE THIS ARTICLE Bedow, J., Mascot,

E. & Morr, D.K. Emergence and manipulation of non-equilibrium Yu-Shiba-Rusinov states. _Commun Phys_ 5, 281 (2022). https://doi.org/10.1038/s42005-022-01050-7 Download citation *

Received: 01 December 2021 * Accepted: 19 October 2022 * Published: 12 November 2022 * DOI: https://doi.org/10.1038/s42005-022-01050-7 SHARE THIS ARTICLE Anyone you share the following link

with will be able to read this content: Get shareable link Sorry, a shareable link is not currently available for this article. Copy to clipboard Provided by the Springer Nature SharedIt

content-sharing initiative